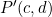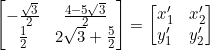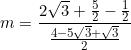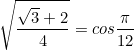Solve  for
for 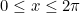
Sine is positive in the first and second quadrants.
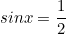
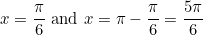
But what if we aren’t given a domain for the  values?
values?
Then we need to give general solutions.
For example,
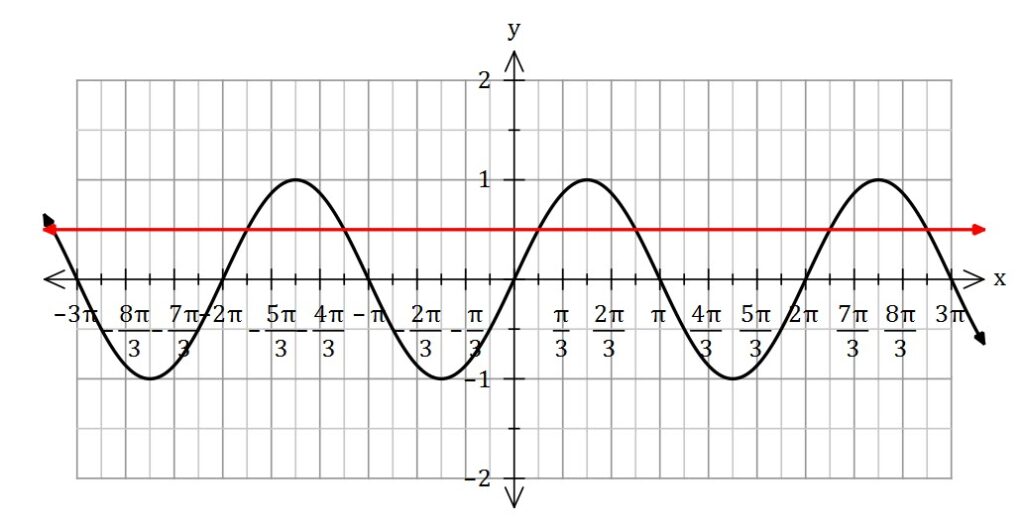
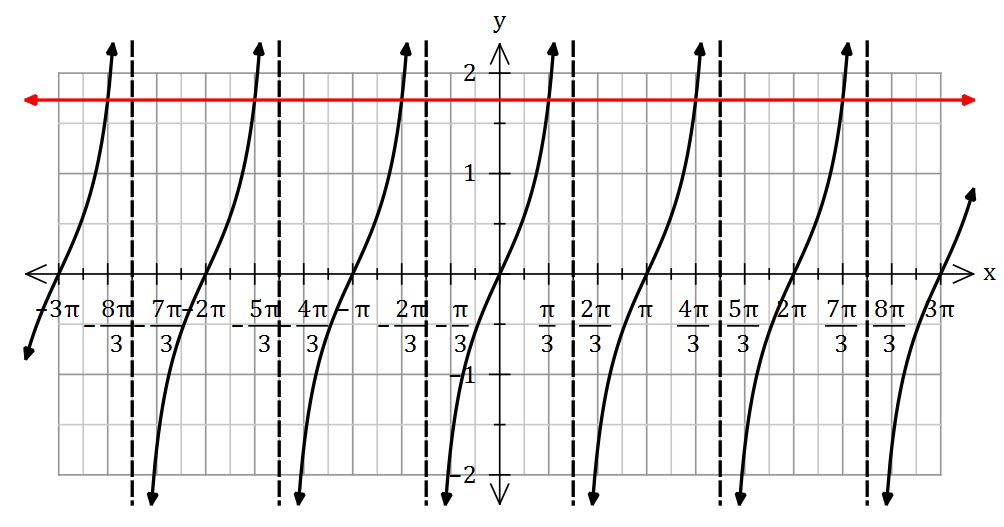
Solve 
As you can see from the sketch above, there are infinite solutions.
The sine function has a period of 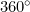 , and so if
, and so if 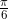 is a solution then
is a solution then 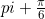 is also a solution. This means
is also a solution. This means 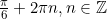 is a general solution. And we can do the same for the second solution
is a general solution. And we can do the same for the second solution 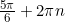 .
.
In general
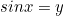
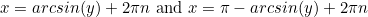
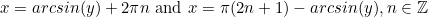
We can turn this into one equation
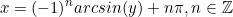
What about cosine?
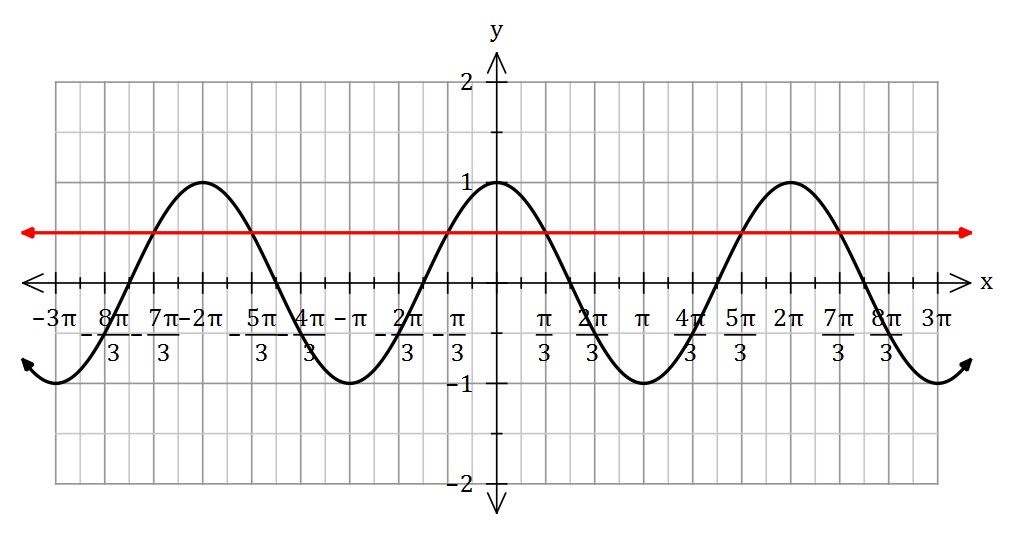
Solve 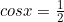
Cosine is positive in the first and fourth quadrants (it also has a period of 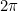 . The first two (positive) solutions are
. The first two (positive) solutions are 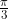 and
and 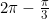 .
.
To generalise, 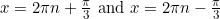 , which we can make into one equation
, which we can make into one equation 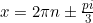
In general
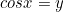
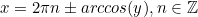
What about the tangent function? Remember tan has a period of 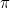 .
.
Solve 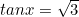
First, note that the solutions are all a common distance (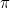 ) apart.
) apart.
Tan is positive in the first and the third quadrant
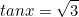
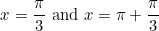
Because all of the solutions are 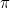 radians apart, the general solution is
radians apart, the general solution is 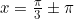
In general
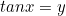

Examples
Solve for all values of  ,
, 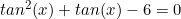
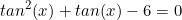
This is a quadratic equation – we need two numbers that add to  and multiple to
and multiple to  ,
, 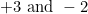
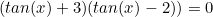
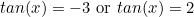
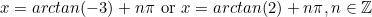
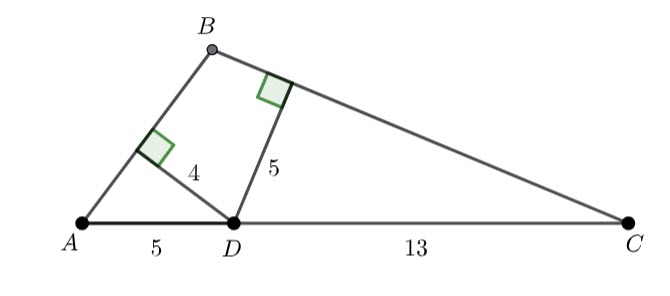
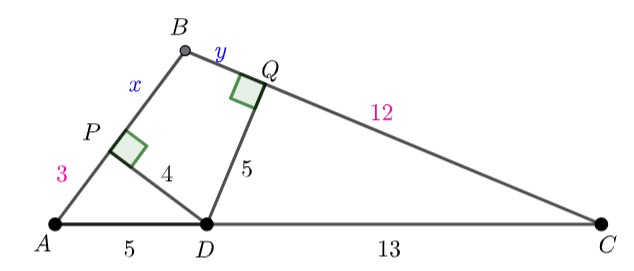
Solve 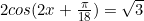

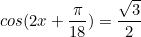
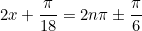
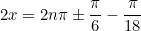
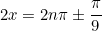
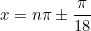
![]()
![]() .
.![]()
![]()
![]() and
and ![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() ,
, ![]()