Category Archives: Quadratics

October 9, 2025 · 6:37 am

Trigonometric Exact Values

Find exactly $sin(18^\circ)$

We must be able to find an arithmetic combination of the exact values we knew to find $18$ .

$\begin{equation*}90=5\times 18\end{equation}$

$\begin{equation*}90-3(18)=2(18)\end{equation}$

I re-arranged as above, so I could take advantage of $cos(90)=0$ and $sin(90)=1$

cos(2x)=cos^2(x)-sin^2(x)=1-2sin^2(x)=2cos^2(x)-1

$\begin{equation*}sin(90-3(18))=sin(2(18))\end{equation}$

$\begin{equation*}sin(90)cos(3(18))-sin(3(18))cos(90)=2sin(18)cos(18)\end{equation}$

$\begin{equation*}cos(3(18))=2sin(18)cos(18)\end{equation}$

$\begin{equation*}4cos^3(18)-3cos(18)=2sin(18)cos(18)\end{equation}$

$\begin{equation*}4cos^3(18)-3cos(18)-2sin(18)cos(18)=0\end{equation}$

$\begin{equation*}cos(18)(4cos^2(18)-3-2sin(18))=0\end{equation}$

Hence,

$\begin{equation*}4cos^2(18)-2sin(18)-3=0\end{equation}$

$\begin{equation*}4-4sin^2(18)-2sin(18)-3=0\end{equation}$

$\begin{equation*}-4sin^2(18)-2sin(18)+1=0\end{equation}$

Use the quadratic equation formula

$\begin{equation*}sin(18)=\frac{-b\pm \sqrt{b^2-4ac}}{2a}\end{equation}$

$\begin{equation*}sin(18)=\frac{2 \pm \sqrt{4-4(-4)(1)}}{-8}\end{equation}$

$\begin{equation*}sin(18)=\frac{2 \pm \sqrt{20}}{-8}\end{equation}$

$\begin{equation*}sin(18)=\frac{-2 \mp 2\sqrt{5}}{8}\end{equation}$

$\begin{equation*}sin(18)=\frac{-1 \mp \sqrt{5}}{4}\end{equation}$

As $sin(18)>0$ , $sin(18)=\frac{-1+\sqrt{5}}{4}$

Leave a Comment

Filed under Addition and Subtraction Identities, Algebra, Identities, Quadratic, Quadratics, Solving, Solving Equations, Solving Trig Equations, Trigonometry, Year 11 Specialist Mathematics

Tagged as exact values, sin(18), trig exact values, trigonometric identities

July 1, 2025 · 10:35 am

Synthetic Division (for factorising and/or solving polynomials)

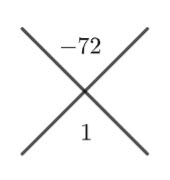
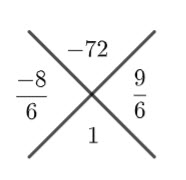
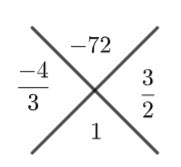
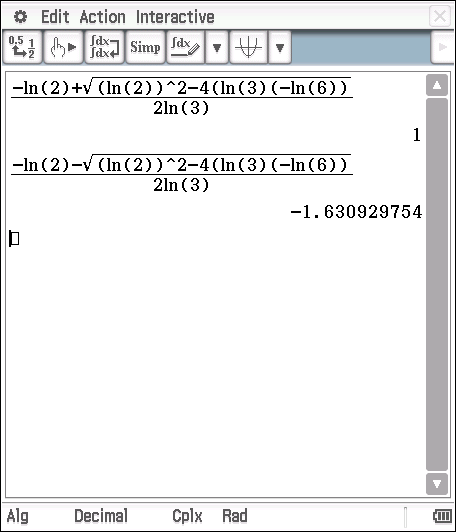
I use synthetic division to factorise polynomials with a degree greater than 2. For example, $f(x)=x^3+2x^2-5x-6$

It works best with monic polynomials but can be adapted to non-monic ones (see example below).

The only problem is that you need to find a root to start.

Try the factors of $-6$ i.e. $(-1, 1, 2, -2, 3, -3, 6, -6)$

$f(-1)=(-1)^3+2(-1)^2-5(-1)-6=0$

Hence, $x=-1$ is a root and $(x+1)$ is a factor of the polynomial.

Set up as follows

Bring the first number down

Multiply by the root and place under the second co-efficient

Add down

Repeat the process

The numbers at the bottom $(1, 1, -6)$ are the coefficients of the polynomial factor.

We now know $x^3+2x^2-5x-6=(x+1)(x^2+x-6)$ .

We can factorise the quadratic in the usual way.

$x^2+x-6=(x+3)(x-2)$

Hence $x^3+2x^2-5x-6=(x+1)(x+3)(x-2)$ .

Let’s try a non-monic example

Factorise $6x^4+39x^3+91x^2+89x+30$

I know $-2$ is a root. Otherwise I would try the factors of 30.

Use synthetic division

Because this was non-monic we need to divide our new co-efficients $(6, 27, 37, 15)$ by 6 (the co-efficient of the $x^4$ term)

$x^3+\frac{9}{2}x^2+\frac{37}{6}+\frac{5}{2}$

We now need to go again. I know that $\frac{-3}{2}$ is a root and $(2x+3)$ is a factor.

Our quadratic factor is $x^2+3x+5/3$ , which is $3x^2+9x+5$ .

The quadratic factor doesn’t have integer factors so,

$6x^4+39x^3+91x^2+89x+30=(x+2)(2x+3)(3x^2+9x+5)$

I think this is much quicker than polynomial long division.

Leave a Comment

Filed under Algebra, Factorising, Factorising, Fractions, Polynomials, Quadratic, Simplifying fractions, Solving Equations

Tagged as non monic synthetic division, Synthetic division

April 30, 2025 · 6:08 am

Factorising Non-Monic Quadratics

The general equation of a quadratic is $ax^2+bx+c$

Let’s explore different methods of factorising a non-monic quadratic (the $a$ term is not $1$ )

Factorise $6x^2+x-12$

We need to find two numbers that add to $1$ and multiply to $-72$ (i.e. add to $b$ and multiply to $a\times c)$

The two numbers are $9$ and $-8$

Method 1 – Splitting the middle term

This is the method I teach the most often

$\begin{equation*}6x^2+x-12\end{equation}$

Split the middle term (the $b$ term) into the two numbers

$\begin{equation*}6x^2-8x+9x-12\end{equation}$

The order doesn’t matter.

Find a common factor for the first term terms, and then for the last two terms.

$\begin{equation*}2x(3x-4)+3(3x-4)\end{equation}$

There is a common factor of $(3x-4)$ , factor it out.

$\begin{equation*}(3x-4)(2x+3)\end{equation}$

Method two – Fraction

$\begin{equation*}6x^2+x-12\end{equation}$

Put $6x$ into both factors and divide by $6$

$\begin{equation*}\frac{(6x-8)(6x+9)}{6}\end{equation}$

Factorise

$\begin{equation*}\frac{2(3x-4)3(2x+3)}{6}\end{equation}$

$\begin{equation*}\frac{6(3x-4)(2x+3)}{6}\end{equation}$

$\begin{equation*}(3x-4)(2x+3)\end{equation}$

Method 3 – Monic to non-monic

$\begin{equation*}y=6x^2+x-12\end{equation}$

Multiply both sides of the equation by $a$

$\begin{equation*}6y=6(6x^2+x-12)\end{equation}$

$\begin{equation*}6y=6^2x^2+6x-72\end{equation}$

$\begin{equation*}6y=(6x)^2+6x-72\end{equation}$

Let $A=6x$

$\begin{equation*}6y=A^2+A-72\end{equation}$

Factorise

$\begin{equation*}6y=(A+9)(A-8)\end{equation}$

Replace the $A$ with $6x$

$\begin{equation*}6y=(6x+9)(6x-8)\end{equation}$

$\begin{equation*}6y=3(2x+3)2(3x-4)\end{equation}$

$\begin{equation*}6y=6(2x+3)(3x-4)\end{equation}$

$\begin{equation*}y=(2x+3)(3x-4)\end{equation}$

Method 4 – Cross Method

$\begin{equation*}6x^2+x-12\end{equation}$

Place the two numbers in the cross

Place the two numbers that add to $1$ and multiply to $-72$ in the other parts of the cross.

Divide these two numbers by $6$ (i.e $a$ )

Simplify

Hence,

$\begin{equation*}(x-\frac{4}{3})(x+\frac{3}{2})\end{equation}$

Which is

$\begin{equation*}(3x-4)(2x+3)\end{equation}$

Method 5 – By Inspection

This is my least favourite method – although students get better with practice

$\begin{equation*}6x^2+x-12\end{equation}$

The factors of $a$ are $1, 2, 3,$ and $6$ and the factors of $12$ are $1, 2, 3, 4, 6, 12$

We know one number is positive and one number negative.

Which give us all of these possibilities

$\begin{equation*}(2x+3)(3x-4)\end{equation}$

With a bit of practice you don’t need to check all of the possibilties, but I find students struggle with this method.

Method 6 – Grid

$\begin{equation*}6x^2+x-12\end{equation}$

Create a grid like the one below


	$6x^2$
		$-12$

Find the two numbers that multiply to $-72$ and add to $1$ and place them in the other grid spots (see below)


	$6x^2$	$-8x$
	$9x$	$-12$

Find the HCF (highest common factor) of each row and put in the first column.

Row $1$ HCF= $2x$ , Row $2$ HCF= $3$


$2x$	$6x^2$	$-8x$
$3$	$9x$	$-12$

For the columns, calculate what is required to multiple the HCF to get the table entry.

For example, what do you need to multiple $2x$ and $3$ by to get $6x^2$ and $9x$ ? In this case it is $3x$ . It’s always going to be the same thing, so just use one value to calculate it,

	$3x$	$-4$
$2x$	$6x^2$	$-8x$
$3$	$9x$	$-12$

The factors are column $1$ and row $1$
$(2x+3)(3x-4)$

The two methods I use the most are splitting the middle term, and the cross method, but I can see value in the grid method.

Leave a Comment

Filed under Algebra, Factorising, Factorising, Polynomials, Quadratic, Quadratics

Tagged as Factorising, factorising non-monic trinomials, non-monic quadratics

April 23, 2025 · 9:30 am

Problem Solving

I am came across this problem and was fascinated. It’s from this book

At first I went straight to the 14-sided polygon, and tried to draw the diamonds (parallelograms), but then I thought let’s start smaller and see if there is a pattern.

Clearly a square contains 1 diamond (itself).

Pentagon

It’s not possible with a pentagon.

Hexagon

A hexagon has 6 diamonds

Septagon

I am guessing it’s not possible to fill a regular 7-sided shape with diamonds

It’s not possible with odd numbers of sides. Regular polygons with an odd number of sides have no parallel sides, so we can’t cover it with rhombi (which have opposite sides parallel).

Octagon

An octagon has 6 diamonds.

We know a decoagon has 10 diamonds (from the question)

Let’s put together what we know

$n$	$4$	$6$	$8$	$10$
Diamonds	$1$	$3$	$6$	$10$

These are the triangular numbers, so when $n=12$ the number of diamonds is $15$ , and for $n=14$ it’s $21$ .

We can work out a rule for calculating the number of diamonds given the number of sides.

Because the difference in the $n$ values is not $1$ , I am going to get $n$ and $D$ in terms of $k$ and then combine the two equations.

From the above table, $n=2k+2$

We know this rule is quadratic as the second difference is constant, hence

$D=\frac{1}{2}k^2+bk+c$

$\begin{equation*}1=\frac{1}{2}+b+c\end{equation}$

(1) $\begin{equation*}\frac{1}{2}=b+c\end{equation*}$

$\begin{equation*}3=\frac{1}{2}2^2+2b+c\end{equation}$

(2) $\begin{equation*}1=2b+c\end{equation*}$

Solve simultaneously, subtract equation $1$ from equation $2$

(3) $\begin{equation*}\frac{1}{2}=b\end{equation*}$

Substitute for $b=\frac{1}{2}$ into equation $1$

$\begin{equation*}\frac{1}{2}=\frac{1}{2}+c\end{equation}$

$c=0$ , therefore $D=\frac{1}{2}k^2+\frac{1}{2}k$

We know $n=2k+2$ hence $k=\frac{n-2}{2}$

Hence $D=\frac{1}{2}(\frac{n-2}{2})^2+\frac{1}{2}(\frac{n-2}{2})$

$D=\frac{1}{8}(n^2-4n+4)+\frac{1}{4}(n-2)=\frac{n^2}{8}-\frac{n}{2}+\frac{1}{2}+\frac{n}{4}-\frac{1}{2}=\frac{n^2}{8}-\frac{n}{4}$

$\begin{equation*}D=\frac{n^2}{8}-\frac{n}{4}\end{equation}$

Let’s test our rule for $n=14$

$\begin{equation*}D=\frac{14^2}{8}-\frac{14}{4}=\frac{49}{2}-\frac{7}{2}=\frac{42}{2}=21\end{equation}$

Leave a Comment

Filed under Area, Geometry, Interesting Mathematics, Puzzles, Quadratics

Tagged as Finding a quadratic rule, regular polygons, rhombus, tessellations

March 31, 2025 · 7:14 am

Deriving the Quadratic Equation formula

My year 10 students have been learning how to complete the square with the idea of then deriving the quadratic equation formula.

The general equation for a quadratic is $y=ax^2+bx+c$

Completing the square,

$\begin{equation*}ax^2+bx+c\end{equation}$

Factorise out the leading coefficient (i.e. $a$ )

$\begin{equation*}a(x^2+\frac{bx}{a}+\frac{c}{a})\end{equation}$

Half the second term (i.e $\frac{b}{a}$ ) and subtract the square of the second term.

$\begin{equation*}a((x+\frac{b}{2a})^2-(\frac{b}{2a})^2+\frac{c}{a})\end{equation}$

$\begin{equation*}a((x+\frac{b}{2a})^2-\frac{b^2}{4a^2}+\frac{c}{a})\end{equation}$

Simplify

$\begin{equation*}a((x+\frac{b}{2a})^2-\frac{b^2}{4a^2}+\frac{4ac}{4a^2})\end{equation}$

$\begin{equation*}a((x+\frac{b}{2a})^2+\frac{-b^2+4ac}{4a^2})\end{equation}$

$\begin{equation*}a(x+\frac{b}{2a})^2+\frac{-b^2+4ac}{4a}\end{equation}$

Now let’s solve

$\begin{equation*}a(x+\frac{b}{2a})^2+\frac{-b^2+4ac}{4a}=0\end{equation}$

$\begin{equation*}a(x+\frac{b}{2a})^2=\frac{b^2-4ac}{4a}\end{equation}$

$\begin{equation*}(x+\frac{b}{2a})^2=\frac{b^2-4ac}{4a^2}\end{equation}$

$\begin{equation*}(x+\frac{b}{2a})=\pm \sqrt{\frac{b^2-4ac}{4a^2}}\end{equation}$

$\begin{equation*}(x+\frac{b}{2a})=\frac{\pm \sqrt{b^2-4ac}}{2a}\end{equation}$

$\begin{equation*}x=-\frac{b}{2a}\frac{\pm \sqrt{b^2-4ac}}{2a}\end{equation}$

Which is the quadratic equation formula.

1 Comment

Filed under Algebra, Quadratic, Quadratics, Solving, Solving, Solving Equations

Tagged as completing the square, quadratic equation formula

November 29, 2024 · 8:11 am

Interesting Equation

I think this one is doing the rounds, I first saw it here.

$\begin{equation*}2^x3^{x^2}=6\end{equation}$

$x=1$ is the obvious answer, $2^1\times 3^1=6$ , but are there more answers?

This was my approach

$\begin{equation*}ln(2^x3^{x^2})=ln(6)\end{equation}$

$\begin{equation*}ln(2^x)+ln(3^{x^2})=ln(6)\end{equation}$

$\begin{equation*}xln(2)+x^2ln(3)-ln(6)=0\end{equation}$

$\begin{equation*}ln(3)x^2+ln(2)x-ln(6)=0\end{equation}$

A quadratic equation.

Hence,

$\begin{equation*}x=\frac{-ln(2)\pm\sqrt{(ln(2))^2-4(ln(3))(ln(6))}}{2ln(3)}\end{equation}$

I then used my calculator

Hence $x=1$ 0r $x=-1.631$

Leave a Comment

Filed under Algebra, Index Laws, Interesting Mathematics, Quadratics, Solving

Tagged as exponentials, index laws, Interesting maths, quadratic solving

May 26, 2024 · 1:07 pm

Quadratic Rule from a Table of Values

How do you find a quadratic rule from a table of values?

For example,

$x$	1	2	3	4
$y$	0	6	14	24

Find the first difference

First Difference

6-0=6

14-6=8

24-14=10

Find the second difference (if the second difference is a constant, then it is quadratic)

Second Difference

8-6=2

10-8=2

The general equation of a quadratic is $y=ax^2+bx+c$

The second difference is $2a$

Hence our equation is now $y=x^2+bx+c$

The $c$ value is the vertical intercept ( $x=0$ ). We can back track in the table

$x$	0	1	2	3	4
$y$	$c$	0	6	14	24

As the first differences are 6, 8, 10, the one between 0 and 1 must be 4

$0-c=4$

$c=-4$

Our equation is now $y=x^2+bx-4$ .

We can now use any other point to find the $b$ value.

Let’s use the point $(2, 6)$

$6=2^2+b(2)-4$

$6=4-2b-4$

$6=2b$

$b=3$

The function is $y=x^2+3x-4$

Let’s try another one

$x$	3	4	5	6
$y$	7	17	31	49

First differences

First difference

Second difference

Second Difference

Hence $2a=4$ , therefore $a=2$

The equation is now $y=2x^2+bx+c$

Instead of back tracking, this time I am going to use two points and simultaneous equations.

Using points $(3, 7)$ and $(4, 17)$

$7=2(3)^2+b(3)+c$

(1) $\begin{equation*}3b+c=-11\end{equation*}$

$17=2(4)^2+b(4)+c$

(2) $\begin{equation*}4b+c=-15\end{equation*}$

Equation 2 – Equation 1

$b=-4$

Substitute $b=-4$ into equation 1

$3(-4)+c=-11$

$-12+c=-11$

$c=1$

Hence the equation is $y=2x^2-4x+1$

Leave a Comment

Filed under Quadratics, Uncategorized

Tagged as Finding a quadratic rule, quadratic rules

May 9, 2024 · 6:08 am

Intersections of Lines and Circles

The three possibilities: no intersection, one point of intersection, or two points of intersection

Once my students have studied circle and quadratic equations, I like to introduce lines intersecting with circles. It tests their quadratic equation solving skills, and if they can use the discriminant.

Example 1

Calculate the point(s) of intersection of

$3x+2y-6=0\ \textnormal{and}\ (x+4)^2+(y+2)^2=9$

Rearrange the line equation

$y=\frac{6-3x}{2}$

$y=3-\frac{3x}{2}$

Substitute for y into the circle equation

$(x+4)^2+(3-\frac{3x}{2}+2)^2=9$

$(x+4)^2+(5-\frac{3x}{2})^2=9$

$x^2+8x+16+25-15x+\frac{9x^2}{4}-9=0$

$\frac{13x^2}{4}-7x+32=0$

$13x^2-28x+128=0$

At this point I like to check the discrimnant

$\Delta=b^2-4ac$

$\Delta=(-28)^2-4\times13\times128$

$\Delta=-5872$

As the discriminate is less than zero, there are no points of intersection.

Example 2

Calculate the point(s) of intersection of

$y=2x+1\ \textnormal{and}\ (x+5)^2+(y+3)^2=16$

$(x+5)^2+(2x+1+3)^2=16$

$(x+5)^2+(2x+4)^2=16$

$x^2+10x+25+4x^2+16x+16-16=0$

$5x^2+26x+25=0$

Check the discriminant

$\Delta=26^2-4\times5\times25$

$\Delta=176$

Therefore there are two points of intersection

$x=\frac{-b\pm\sqrt{b^2-4ac}}{2a}$

$x=\frac{-26\pm\sqrt{176}}{10}$

$x=\frac{-26\pm4\sqrt{11}}{10}$

$x=\frac{-13\pm2\sqrt{11}}{5}$

Substitute x into y

$y=2(\frac{-13\pm2\sqrt{11}}{5})+1$

$\textnormal{The two points are}\ (\frac{-13+2\sqrt{11}}{5},\frac{-21+4\sqrt{11}}{5})$

$\textnormal{and}\ (\frac{-13-2\sqrt{11}}{5},\frac{-21-4\sqrt{11}}{5})$

Example 3

Find the value of k so that the line and the circle intersect at one point (i.e. the line is a tangent to the circle)

$-kx+y-5=0\ \textnormal{and}\ (x-2)^2+(y-3)^2=\frac{36}{5}$

$y=kx+5$

$(x-2)^2+(kx+5-3)^2=\frac{36}{5}$

$(x-2)^2+(kx+2)^2=\frac{36}{5}$

$x^2-4x+4+k^2x^2+4kx+4-\frac{36}{5}=0$

$(1+k^2)x^2+(4k-4)x+\frac{4}{5}=0$

Find the discriminant (remember for one solution we want the discriminant to be zero)

$\Delta=b^2-4ac$

$\Delta=(4k-4)^2-4\times(1+k^2)\times\frac{4}{5}$

$\Delta=16k^2-32k+16-\frac{16}{5}-\frac{16k^2}{5}$

$0=16k^2-32k+16-\frac{16}{5}-\frac{16k^2}{5} \[0=80k^2-160k+80-16-16k^2$

$0=64k^2-160k+64$

$0=32(2k^2-5k+2)$

$0=2k^2-5k+2$

$0=2k^2-4k-k+2$

$0=2k(k-2)-1(k-2)$

$0=(2k-1)(k-2)$

$k=\frac{1}{2}\ \textnormal{and}\ k=2$

Leave a Comment

Filed under Circles and Quadratics, Quadratics

Tagged as discriminant, intersection of circles and lines, quadratics

April 2, 2024 · 7:10 am

Factorising Non-Monic Trinomials

I factorise trinomials by using the grouping method, I will show you what I mean

But one of my students showed me a different way

I have put in more steps than is necessary. I think this might be a quicker method.

Leave a Comment

Filed under Factorising, Quadratics

Tagged as factorising non-monic trinomials, factorising trinomials

Possible factorisations	$b$ term of expansion
$(x-1)(6x+12)$	$12x-6x=6x$	No
$(x-2)(6x+6)$	$6x-12x=-6x$	No
$(x-3)(6x+4)$	$4x-18x-14x$	No
$(2x-1)(3x+12)$	$24x-3x=21x$	No
$(2x-2)(3x+6)$	$12x-6x=6x$	No
$(2x-3)(3x+4)$	$8x-9x=-1x$	Almost, switch the signs
$(2x+3)((3x-4)$	$-8x+9x=1x$	Yes

Category Archives: Quadratics

Trigonometric Exact Values

Synthetic Division (for factorising and/or solving polynomials)

Factorising Non-Monic Quadratics

Method 1 – Splitting the middle term

Method two – Fraction

Method 3 – Monic to non-monic

Method 4 – Cross Method

Method 5 – By Inspection

Method 6 – Grid

Problem Solving

Pentagon

Hexagon

Septagon

Octagon

Deriving the Quadratic Equation formula

Interesting Equation

Quadratic Rule from a Table of Values

Intersections of Lines and Circles

Example 1

Example 2

Example 3

Factorising Non-Monic Trinomials

Recent Posts

Categories

Archives