Tag Archives: area of triangles

April 21, 2025 · 11:08 am

Area/Geometry Problem

This problem is from The Geometry Forum Problem of the Week June 1996

In triangle ABC, AC=18 and D is the point on AC for which AD=5. Perpendiculars drawn from D to AB and CB have lengths of 4 and 5 respectively. What is the area of triangle ABC?

I put together a diagram (in Geogebra)

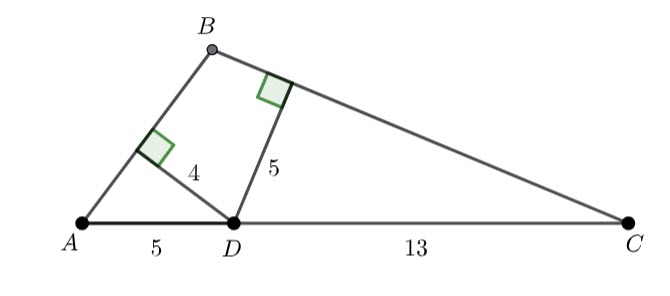
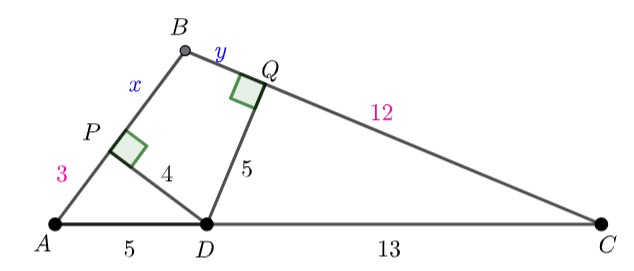
Add points P and Q

Triangle APD and triangle DQC are right angled. Using pythagoras, $AP=3$ and $QC=12$

$BQDP$ is a cyclic quadrilateral and $BD$ is the diameter. I am not sure if this is useful, but it is good to notice.

$\begin{equation*}sin(A+B+C)=sin(180)=0\end{equation}$

$\begin{equation*}sin((A+C)+B)=sin(A+C)cosB+sinBcos(A+C)=0\end{equation}$

$\begin{equation*}cosB(sinAcosC+sinCcosA)+sinB(cosAcosC-sinAsinC)=0\end{equation}$

$\begin{equation*}cosB(\frac{4}{5}\times\frac{12}{13}+\frac{5}{13}\times\frac{3}{5})+sinB(\frac{3}{5}\times\frac{12}{13}-\frac{4}{5}\times\frac{5}{13})=0\end{equation}$

$\begin{equation*}cosB(\frac{48}{65}+\frac{15}{65})+sinB(\frac{36}{65}-\frac{20}{65})=0\end{equation}$

$\begin{equation*}\frac{63}{65}cosB+\frac{16}{65}sinB=0\end{equation}$

$\begin{equation*}63cosB+16sinB=0\end{equation}$

$\begin{equation*}63+16tanB=0\end{equation}$

$\begin{equation*}tanB=\frac{-63}{16}\end{equation}$

If $tanB=\frac{-63}{16}$ then $sinB=\frac{63}{65}$

Now,

$\begin{equation*}\frac{y+12}{sinA}=\frac{18}{sinB}\end{equation}$

$\begin{equation*}y+12=\frac{4}{5}(18)\frac{65}{63}\end{equation}$

$\begin{equation*}y+12=\frac{104}{7}\end{equation}$

Hence the Area is

$\begin{equation*}A=\frac{1}{2}(18)(\frac{104}{7})sinC\end{equation}$

$\begin{equation*}A=\frac{1}{2}(18)(\frac{104}{7})\frac{5}{13}\end{equation}$

$\begin{equation*}A=\frac{360}{7}=51.43\end{equation}$

Leave a Comment

Filed under Area, Finding an area, Geometry, Identities, Non-Right Trigonometry, Pythagoras, Trigonometry

Tagged as area, area of triangles, problem solving

April 3, 2025 · 7:28 am

Area of Regular Polygons

Finding the area of a regular polygon when you know the side length

Find the area of an $n-$ sided regular polygon if you know the side length, $l$ .

An octagon for a visual reference

Find the $h$ of the triangle in terms of $l$ and theta.

$tan(\theta)=\frac{\frac{l}{2}}{h}$

$h=\frac{l}{2tan(\theta)}$

Remember the area of a triangle is $A=\frac{1}{2}bh$

Hence, $A=\frac{1}{2} l \times \frac{l}{2tan(\theta)}=\frac{l^2}{4tan(\theta)}$

And $\theta=\frac{360}{2n}=\frac{180}{n}$

Therefore $A=\frac{l^2}{4tan(\frac{180}{n})}$

There are $n$ triangles in an $n-$ sided polygon

(1) $\begin{equation*}A=\frac{nl^2}{4tan(\frac{180}{n})}\end{equation*}$

A=\frac{6\times10^2}{4tan(\frac{180}{6})}

A=\frac{600}{4(\frac{1}{\sqrt{3}})}

Finding the area of a polygon if you know the inradius or the apothem

The apothem and the inradius are the same. It is the radius of the incircle.

Find the area of the triangle in terms of $a$ and theta.

$tan(\theta)=\frac{\frac{l}{2}}{a}$

$l=2atan(\theta)$

$A=\frac{1}{2}bh$

$A=\frac{1}{2}2atan(\theta)a=a^2tan(\theta)$

And $\theta=\frac{180}{n}$

Hence for an $n-$ sided polygon

(2) $\begin{equation*}A=na^2tan(\frac{180}{n})\end{equation*}$

A=5\times 4.5^2tan(\frac{180}{5})

A=73.56cm^2

Finding the area of a regular polygon given the circumradius

The circumradius is the radius of the circumscribed circle ( $R$ in the diagram above)

Remember the area of triangle formula

$A=\frac{1}{2}absin(\theta)$

$A=\frac{1}{2}R^2sin(\theta)$

$\theta=\frac{360}{n}$

Hence, $A=\frac{1}{2}R^2sin(\frac{360}{n})$

Hence, for an $n-$ sided polygon

(3) $\begin{equation*}A=\frac{nR^2sin(\frac{360}{n})}{2}\end{equation*}$

A=\frac{8\times 10^2sin(45)}{2}

A=200\sqrt{2}cm^2

Leave a Comment

Filed under Area, Area of Triangles (Sine), Finding an area, Non-Right Trigonometry, Regular Polygons, Right Trigonometry, Year 11 Mathematical Methods

Tagged as area of regular polygons, area of triangles, trig